Motions of the Top, Teetotum and Gyroscope

 

By Edward David Ashe

 

[Originally published by the Literary and Historical Society of Quebec in Transactions, New Series, No. 2 (1864)]

 

(Read before the Socioty, January 6th, 1864.)

 

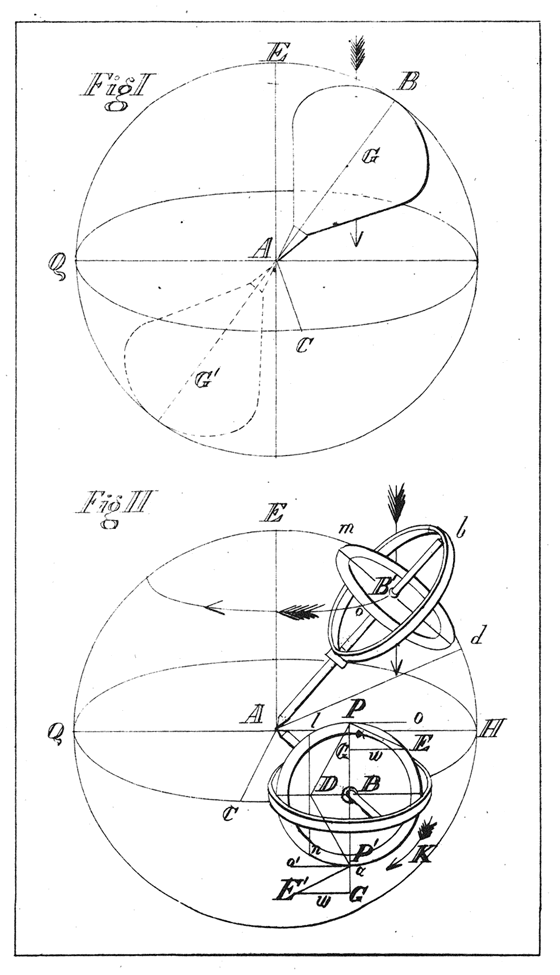
BEFORE entering into a description of the experiments upon the Gyroscope, Top, and Teetotum, and offering a solution of their motions under varying circumstances, I will just answer this question, viz.: What will be the consequence of removing all other atoms excepting those of which the top is composed, from the sphere BCQ, which is supposed to be rotating round the axis AB? (Fig. I.)

 

The effect will be exactly the same as if all the atoms of the rotating sphere remained in their places, and an additional weight, equal to that of the top, were applied as a force passing through G, perpendicular to the horizon ; a force that tends to turn the sphere round the horizontal axis A C. As no one atom drags another, but each atom has a motion corresponding to its position in the sphere, and the force acting upon it, it is evident that we may cither take away the atoms of a corresponding top G', from the opposite hemisphere, or we may double the weight of the top.

 

The number and position of the atoms uncompensated will in either case be the same.

 

Therefore, under these circumstances, we arc allowed to consider the top, teetotum, or gyroscope, as part of a sphere, which is revolving around a common axis, with a similar figure of revolution to the top, teetotum, or gyroscope, taken away from the opposite hemisphere.

It must be thoroughly understood that I consider the revolving body to be a part of a sphere, the point upon which the top, teetotum or gyroscope spins, to be the centre of that sphere, with a similar figure to the revolving body taken away from the opposite hemisphere.

 

Advancing towards the subject with this view, and with the tract of the Astronomer Royal on the "Composition of Rotar Motion" as my text book, all mysteries and inconsistencies disappear

.

Let a gyroscope be placed with its axis horizontal, and the disc PP' in a vertical position, and let the disc revolve from left to right.

 

Here I suppose a sphere, HBQ, revolving round its axis, AB, which is horizontal, from left to right in the direction of the arrow K, and a similar figure to the gyroscope, taken from the opposite hemisphere, or what is the same thing, a weight equal to that of the gyroscope, to be applied as a force, acting in a vertical line PB, passing through the centre of gravity of the gyroscope.

 

This force tends to turn the sphere round the horizontal axis QH.

 

Then by Prop. 1: "If a body revolve about an axis AB with an angular velocity w, and if a force be impressed upon it which would make it revolve about the axis A C, with an angular velocity w', then the body will not revolve about either of the axis AB, AG, but about an axis AD, in the plane BAC; so that sin BAD: sin CAD:: w': w."— (Fig. 2.)

 

And by Prop. 3: “If a uniform force act upon the body, tending to give it a motion of rotation about an axis which is always perpendicular to the axis about which it is at each instant revolving, and always in the plane BAC, then the angular velocity will be unaltered." In this case the axis QH, about which gravity tends to turn the gyroscope, is always horizontal and perpendicular to the axis of the gyroscope AB.

 

And by Prop. 4: "Under the same circumstances the axis of rotation has a uniform motion in space from the position AB towards AC, and the angle described in 1s = a/w”

 

Where a represents the angular velocity round the axis QH' caused by gravity acting on the weight of the gyroscope, and w the velocity of the sphere or gyroscope on its polar axis AB.

 

The axis QH, round which gravity tends to turn the sphere, must always be horizontal, and at the same time always at right angles to the polar axis AB. Consequently, if the velocity round the polar axis w is constant, equal angles to a/w will be added to the to angle BAD each second.

 

But suppose that PDP' represents the disc of a gyroscope, (fig. 2) with its axis AB, horizontal, and suppose that the disc is revolving in the direction of the arrow K.

 

Now take any two points in the disc, such as P, and P', and draw PO, and P'O', to represent, in direction and magnitude, the tangential velocity of those points, caused by the rotation of the disc round the axis AB; and also let PG, and P'G', in a similar manner represent the velocity caused by gravity in turning those points round the horizontal axis QH, in a short interval.

 

Then PE and P’E' will be the resultant of those forces, and the points P and P' will have a tendency to move in those directions at that instant; but as the centre round which the points P and P’ have a tendency to move must be in a line perpendicular to those directions, it follows that the intersection of the lines PD and P’D must be the point round which P and P’ have a tendency to turn at that moment.

 

And taking any other point in the disc, and applying the force of gravity and centrifugal force, it will be found that that point also will have a tendancy to turn round the same point D. Now, as the points in the disc are constrained, they cannot revolve round the point D; but as the atoms on one side of the line In, have too small a centrifugal force for their position, whilst those on the other side of the line, have too great a centrifugal force for their position, it follows that the point B will be urged towards D; Because the triangle PGE is similar to the triangle PBD, we have DB/PB = PG/GE = a/w As PG is a function of AB, (the distance of the centre of gravity of the gyroscope, from the support A,) DB, will represent the angular velocity to the radius AB,—the same fraction as Professor Airy uses  for the angular velocity of the axis AB, towards A C, (Prop. 4.)

 

Now, when the axis of the gyroscope is not horizontal, but in the position Ab, (fig. 2), the force of gravity acts on the point B, in the direction of the arrow ; and as the point B is constrained to turn upon the point A, it has a£ that moment a tendency to move in the direction of the tangent B'd; and resolving the force of gravity in the direction Bd, at right angles to the axis Ab, we have the force in the direction Bd=G cosθ, where G represents the force of gravity, and θ the angle that the axis of the gyroscope makes with the horizon; and as all the points in the line md are constrained at that instant to move in the direction of the tangent, we have, in the first position of the gyroscope, to substitute for gravity (represented by the line PG =θ) G=a cosθ.

Now, the greater the angleθ, the slower the velocity of the gyrascope round AE. We also see that the greater θ is, the smaller is the circle round which B moves, but the velocity of the point B, and the circle round which B moves, varies as cosθ, which accounts for the fact, that whatever position the axis of the gyroscope is placed in, the point B always performs a revolution round the vertical axis AE, in the same time. In this experiment care must be taken that the velocity of the disc on the Polar axis is the same in all cases, i.e., that the fraction a/w is the same.

 

Another peculiarity of the gyroscope is, that when the axis AB is placed in any position, such as AB (fig. 2), after a short time it is clearly seen that the velocity of the point B, round the vertical axis AE, is greater than at first starting, and also that it continues to increase its velocity.

 

The explanation of this, from what has been said before, is very simple.

 

Since we have shown that the angular velocity of the axis AB towards AC is equal to a/w where a/ represents the voloeitv round the horizontal axis (fig. 2), caused by gravity, which is constant; but w/ represents the velocity of the disc on its polar axis, which is always decreasing, we see then that the fraction a/w is continually increasing, therefore larger angles are added each moment to BAD.

 

After the gyroscope has been placed in any position, it is soon seen that the axis AB begins to droop, and it continues to fall until finally the circumference of the gyroscope touches the support H, fig. 3. It is very evident that gravity has nothing whatever to do with pulling down the axis AB, because we see in the 1st Prop, that when the gyroscope is acted on by gravity and the rotation of the sphere, the new position of the axis must be in the plane BAC; and that as the axis AC is of a necessity always horizontal, and at right angles to the polar axis AB, it follows that the axis AC generates a circle, whilst the polar axis AB generates a cone, except when AB is horizontal.

 

We see by the foregoing propositions that the force that causes the axis Ab (fig. 2) to fall must be a force that tends to turn the sphere round the vertical axis AE.

 

Take any point d in the vertical plane EAH, and suppose that the sphere HCQ is revolving round the axis Ab, and let Ad be the new position of the axis, then the velocity round the vertical axis AE in the direction QGH must tend to depress the point d below the surface of the paper, as much as the rotation round the axis of the sphere Ab tends to raise it.

 

From this we see that if a sphere is revolving round its axis Ab in direction dom, and a force be applied to turn the sphere round its vertical axis AE in the direction QCH, then will the axis of the gyroscope Ab assume some lower position, such as Ad.

 

Now, it is easy to discover that the force that tends to turn the gyroscope round the vertical axis AE in the direction QCH is resistance of the air. It has been shown that the slower the disc of the gyroscope revolves on its axis, the greater the fraction a/w or the angular velocity of the polar axis AB round the vertical AE, and consequently the greater is the resistance of the air opposed to the gyroscope, and consequently the axis AB of the gyroscope will droop more rapidly as the fraction a/w increases.

 

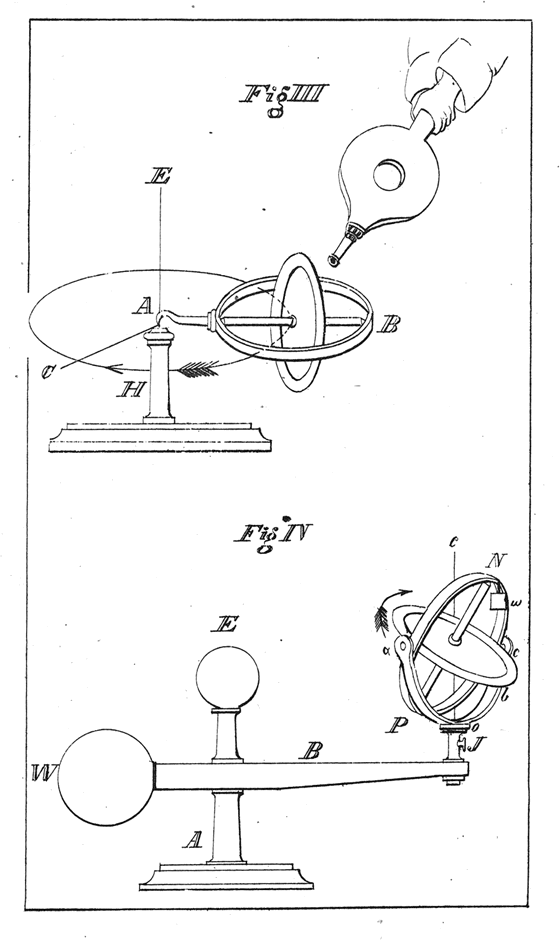
But we will prove by experiment that resistance of the air is the force that causes the axis AB to droop. Place the gyroscope in the position (fig. 3,) with the axis AB horizontal, and the axis moving in the direction BHC.

 

If the resistance of the air causes the axis AB to droop, then if we take a pair of bellows and blow in the direction BH and give the gyroscope a fair wind, then the force of air tends to turn the gyroscope round the vertical axis AE in an opposite direction, and the axis AB, instead of drooping, will immediately begin to rise; which it does, and the harder you blow the quicker the axis will rise. In order that you may not suppose that you are blowing the gyroscope up, hold the bellows a little above the gyroscope, and the effect will be that the part of the force that tends to depress the gyroscope, or to turn the sphere round the horizontal axis AC, will assist gravity and quicken the velocity of AB towards AC.

 

If you blow from beneath sufficiently strong so that you overcome the force of gravity, then the motion of the gyroscope round the vertical axis AE immediately stops.

 

In a gyroscope fitted with a counter weight W, and the support made to turn on an axis AE, fig. 4. The gyroscope is fitted with the steel shaft o, of the outer frame b, to move freely in the pipe J.

 

When the disc was revolving in the direction of the arrow, and when I turned the arm of the support B rapidly round the axis AE, the pole N the gyroscope would droop.

 

Now, resistance of the air could have nothing to do in turning the sphere NP round the vertical axis o e, for in this form of gyroscope, the centre of gravity of the disc is supported by the steel shaft O, and therefore some other force must tend to turn the sphere round the vertical axis o e, as it is only by such a force that the pole is made to fall.

 

But in this case, the friction of the pipe against the steel shaft is the force that tends to turn the gyroscope round the vertical axis in an opposite direction to that in which the arm of the support B is moving.

 

For, as the axis of the gyroscope FN remains in one direction, whilst the arm of the support B is turned round a whole circle, it follows that the pipe J is rubbed against the shaft o, during that time; but to prove that the friction of the pipe is the force that causes the pole N to droop, I moved the arm B rapidly in the opposite direction, changing thereby the direction of friction round the vertical axis o e, and immediately the pole N began to rise.

 

Another form of the same experiment is seen by hanging a small weight to the ring that supports the axis of the gyroscope. Now, as this weight exerts a force to turn the sphere (which the gyroscope represents) round the horizontal axis a c, the processional motion takes place, getting quicker and quicker as the rotation of the disc on its polar axis PN gets slower, or the fraction a/w gets larger.

 

The pole begins to droop as in the former experiment, in consequence of the friction of the pipe against the shaft o, which may be considered as a force tending to turn the sphere round the vertical axis in an opposite direction to that in which the weight w is moving.

 

If you stop the precessional motion by turning a screw in the pipe J, the weight w falls very rapidly, in consequence of the greater force being exerted to turn the. gyroscope round the vertical axis, which then is exactly equal and opposite to the force that moves the sphere round the axis o e.

 

Now, take the case where the gyroscope is attached to a string. (fig. 5.)

 

Of course it is immaterial whether the centre of the sphere, or the end of the axis of the gyroscope, is supported on a point, or sustained by a string; the direction of the force is the same in both cases.

 

When the sphere or disc revolves on its polar axis in the direction of the arrow, the axis AB begins to revolve round the vertical axis, or string in the direction BD, and the moment it does so there is a centrifugal force= V2/r where V the velocity in the curve, and r the radius of curvature, and in consequence of this centrifugal force the string leaves the vertical position, making an angleθ.

 

As the centripetal force must always be equal to the centrifugal force, and always in the same line, the point A leaves its original position, taking up some position such as A', until the strain on the string multiplied by sine Q is equal the centrifugal force, that is, P sine θ= V2/r, where P represents the strain in the string. It is very evident that the force in the direction A'O must be equal to the centrifugal force, and that the gyroscope will fly out until it does.

 

The radius of curvature is evidently B'0, as B'0 must be in a straight line, consequently the point A' is carried round in a circle.

 

It must be remembered that no pulling of the string can in any way affect the inclination of the axis A'B' to the horizon.

 

In trying this experiment it is difficult to prevent the whole getting a pendulous motion, which gives the gyroscope the appearance of a bird with a piece of string tied to its leg, but on making due allowance for the different motions, this experiment offers no novelty that cannot be fully explained by the tract on "Rotary Motion."

 

I will now explain why a top goes to sleep, or how it is that the axis of the top assumes a vertical position, and how the centre of gravity of the top is raised through a distance equal to the versine of the angle of inclination.

 

In this case as in others, I consider the top as part of a sphere, the point of support the centre of that sphere, the top and the sphere both revolving on a common axis.

 

When a top is spun, the following peculiarities are observed:

 

If the axis of the top is inclined, the top will describe a curve with the pole of the top, or sphere, leaning in the direction of the normal to the curve. The top assumes a vertical position gradually, and as it does so, the radius of the curve that the top is describing becomes less and lesser, and finally the top goes to sleep with its axis in a vertical position.

 

After a time, when the rotation of the top on its axis is greatly diminished, the peg of the top describes small circles, and the axis of the top inclines more and more towards the horizon, whilst the centre of gravity of the top remains nearly stationary, and the top rotates round the vertical line. When the inclination of the axis of the top gets so great that the bilge of the top touches the ground, it then rolls into the gutter.

 

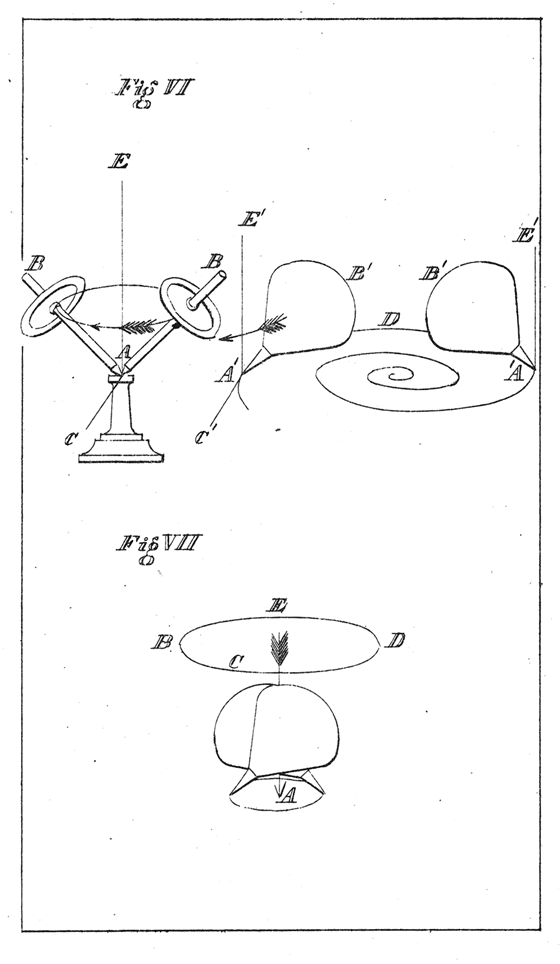
In offering an explanation of these different motions of the top (fig. 6), I may observe that the translation of the centre of the sphere, or the motion of the peg of the top along the curve, does not in the least interfere with the rotation of the top or sphere. [1]

 

The top may be considered a gyroscope, with the centre of the sphere in motion, instead of being fixed as in the gyroscope.

 

The position A'B' of the top corresponds to the position AB of the gyroscope, the same force of gravity tending to turn the top and gyroscope round the horizontal axes A'C AC in the same direction.

 

It follows, from the 1st Prop., that the axis of top and gyroscope must revolve round the vertical axes AE A'E', in the same direction.

 

In the gyroscope the centre of the sphere is kept in one position; in the top the centre of the sphere is free, and as the top inclines the circumference of the peg, rolls along the ground and would describe a straight line, only the force of gravity, tending to turn the top round a horizontal axis, compounded with the rotation of the top on its polar axis, produces a rotation of the axis of the sphere or top, round the vertical axis AE, with an angular velocity

= a/w; Nor does it make any difference (fig. 6) that in the case of the gyroscope the vertical axis AE is stationary, whilst the vertical axis round which the top moves is in motion.

 

The horizontal axis round which gravity tends to turn the top A'C is always a tangent to the curve that the top is at that moment describing.

 

The slower the top revolves on its axis, the greater the fraction a/w, and consequently the shorter the radius of curvature gets.

 

In the gyroscope with the centre of the sphere fixed, as the polar axis revolves round the vertical, in the direction of the arrow, the resistance of the air is opposed to its motion, and, as it has been shewn, tends to make the axis of the gyroscope droop. But in the top, as the centre is free, and moves along the curve in the direction of AD, the resistance of the air acts in a contrary direction, and tends to turn the sphere or top round the vertical axis in the direction of the arrow, which force has been shewn by experiment and by Prop. 1, will cause the axis to rise.

 

As the top gains the vertical position, a smaller circumference of the peg is brought in contact with the ground. This added to the greater velocity of the axis A'B' round the vertical, causes the radius of curvature to diminish and ultimately the top to sleep

 

Should the peg of the top get into a hole before it assumes the vertical position, it will never go to sleep, as the resistence of the air then tends to turn the sphere round the vertical axis in the wrong direction.

 

After the top has remained asleep for some time, the rotation of the top on its axis becomes perceptibly slower, and consequently has not velocity enough to overcome the inequalities of the ground on which it is spinning. The axis of the top is then thrown out of the perpendicular, when gravity instantly acts as a force to turn the sphere round a horizontal axis. But instead of the top starting off on a similar curve to what it did at first, when the axis of the top was equally inclined (fig. 7), it now (in consequence of the a fraction a/w becoming so large) describes a small circle with the centre of gravity stationary on the line AE.

 

As the head of the top is now moving in an opposite direction to what the peg is, resistance of the air again acts in the wrong direction, and tends to turn the sphere round the vertical axis AE in the direction BCD. The fate of the top is now sealed. The slower the rotation of the top on its polar axis becomes, the greater the fraction, a/w and the quicker the rotation of the sphere round the vertical axis AE; consequently, the polar axis droops more quickly than at first, and ultimately the bilge touches the ground and the top rolls away.

 

In thus offering a solution to the motions of the gyroscope, top, and teetotum, I have assumed that the peg of the top, and support of the gyroscope, is the centre of a sphere revolving upon a common axis, with a similar figure of revolution taken away from the opposite hemisphere, and by so doing I can, for every motion of these astronomical toys, point out a proposition in the tract of the Astronomer Royal on rotatory motion, which fully accounts for those motions.

 

Dr. Whewell, in speaking of the top, says: “The axis can never become more nearly vertical than it was at the beginning of the motion, though it will at intervals return to the inclination which it then had. But in the experiment, the top, if inclined at first, will approach to a vertical position, which it will, as near as the senses can judge, attain and preserve for some time, and the centre of gravity will frequently describe a curve approaching to a circle, while the apex or foot of the instrument remains stationary. These differences of theory and practice appear to be attributable to the effects of friction."

 

Euler thus explains the effect of friction in causing a top to raise itself into a vertical position: “The friction will perpetually retard the motion of the apex P of the instrument, and at last reduce it to rest. If this happen before the top fall, it must then be spinning in such a position, that the point can remain stationary. But this cannot be if it be inclined. Hence, it must have a tendency to erect itself into a vertical position."